When it comes to options, one of the most appealing things about them is their payoff structure. Particularly the fact that it caps losses without using a stop loss and leaves room for unlimited profits.
This results in a payoff function that looks like the graph below:
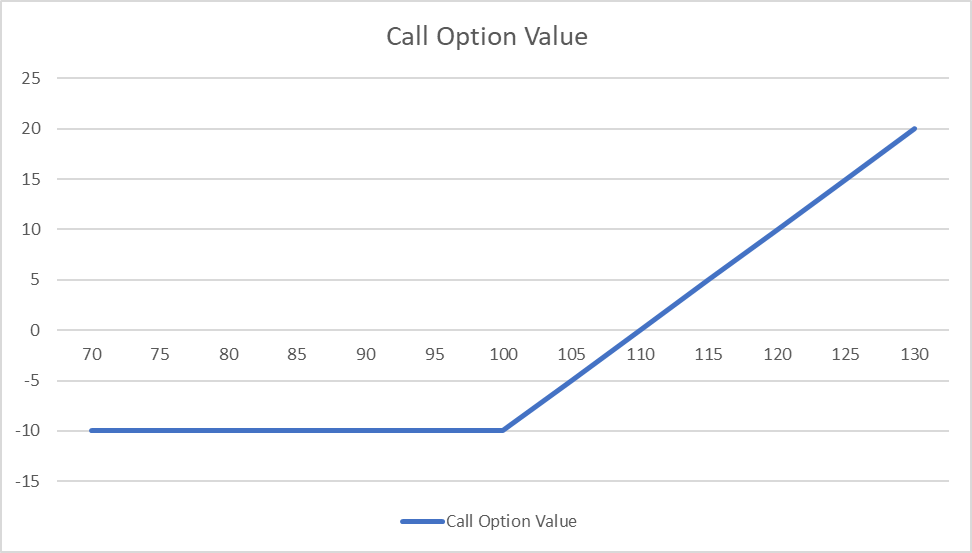
This provides an advantage because, with a payoff structure like this (often referred to as f(x)), you don’t really need to worry about predicting where the asset is going to move to (often referred to as x). Even if it was perfectly normal.
All you need to worry about is whether or not your exposure (or payoff structure, a.k.a, f(x)) has a positive value or negative value when you sum all of the possible outcomes, multiplied by their corresponding probabilities of occurring.
Now in order for investors to be able to take advantage of these options contracts, there needs to be a liquid and robust options market that they can easily and readily interact with.
In order for this to exist, there need to be market makers, whose goal is to provide as much liquidity to the market as possible, in order for it to be a liquid and robust market.
However, in order for them to do this, they need to be able to hedge against these option payoffs using the option’s underlying, otherwise, they wouldn’t be able to stay in business for long.
This is where the concept of Dynamic Hedging comes in.
In its simplest form, Dynamic Hedging is taking a dynamic position in the option’s underlying with the sole purpose of replicating the options payoff structure or f(x).
Let’s walk through a quick example using the call option above.
When an investor buys this option, the person who sold them the option is taking the inverse of this payoff after receiving a credit of $10 from selling the option. Because of this, they need to hedge their exposure to it by taking a dynamic position in the underlying asset of the option.
In this case, they need to start longing the stock and they need to maintain a position that equals the delta of the option, multiplied by the number of shares in the option contract. In our case, this is 100 shares.
Let’s assume this option was at the money and had a delta of .5 at the time it was purchased.
This would mean the seller would immediately need to buy 50 shares of the underlying in order to be hedged.
Then as the underlying increases and decreases in value, so does the option’s delta.
In fact, the delta will increase and decrease in exact proportion to the probability of the option contract finishing in-the-money. This means the delta is the option’s probability of profit and will always be between 0 and 1.
So if the price of the option increases causing the delta of the option to be 0.6, this would mean the option seller needs to buy an additional 10 shares in order to dynamically hedge against the option’s payoff.
If the price then decreases and the delta was now .3, the seller would need to sell back 30 shares in order to once again be dynamically hedged against the option contracts payoff or f(x).
The more granular the option seller is in hedging against the option’s delta, the more accurately the payoff of the position in the underlying will mirror the option contract’s payoff.
If volatility remained constant, the overall PnL of the dynamic hedging position in isolation would equal the credit received when the option contract was sold which is $10 in our case.
If volatility increased, the cost of dynamic hedging would increase. If volatility decreased, the cost of the dynamic hedge would decrease with it.
Below is an image showcasing this with an option that was sold when IV was at 25%.
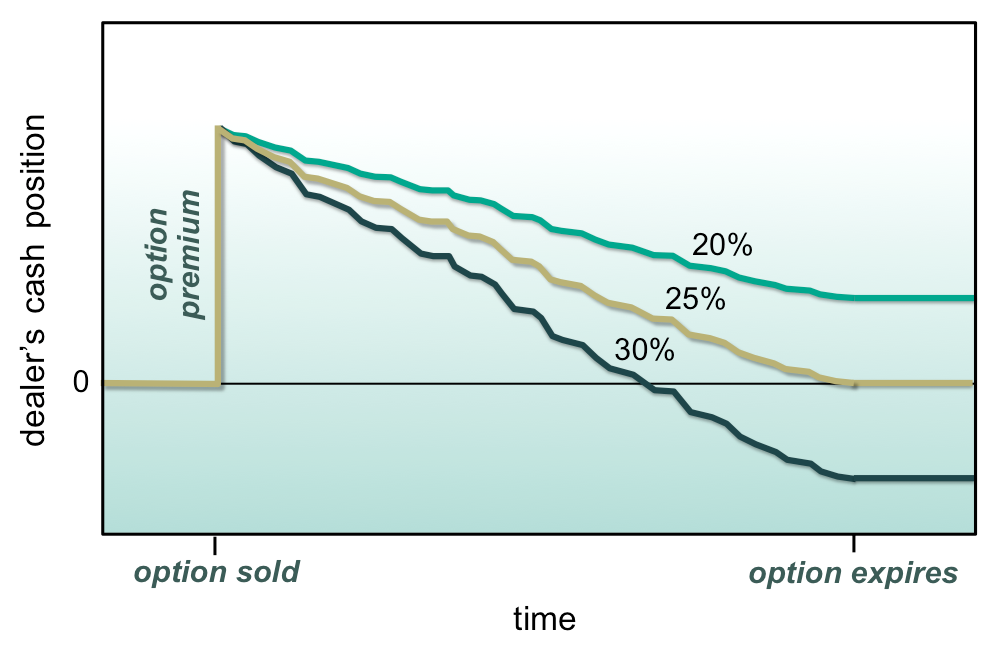
This is truly facinating because what it means is, at the limit, the premium collected from selling an option, combined with the PnL of dynamically hedging it through its life, will exactly equal the option’s payoff for any path it takes.
And in aggregate, when you do this thousands of times, the average PnL of all your dynamic hedging, will be $0, assuming all things remained equal.
Again, this is in aggregate. Remember, you will use the premium collected to help fulfill the payoff deserved by the option buyer PLUS the gamma PnL. So inaggregate of all the options you sell, you will give back all the premium collected, and what you keep is the average result of all your dynamic hedging.
The result of dynamic hedging is what really determines the profitability of a market maker.
If you underpriced your options, the average PnL of all your dynamic hedging would be negative, because you sold the option for less than it’s worth.
If you overpriced the option, and were able to find willing buyers, the average PnL of all your dynamic hedging would positive, because you were able to sell it for more than its worth.
By being able to replicate this payoff or f(x), you no longer need to worry about x in a directional sense. You simply just want x to be volatile and you let your f(x) do the work.